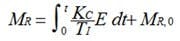The previous two articles ("Neglect Level Control at Your Peril" and "Be Levelheaded About Surge-Tank Control") examined issues pertaining to proportional-integral (PI) control of vessel level. Especially for surge vessels, low values of the controller gain are appropriate. This raises two issues:
1. To avoid cycles in the level, reset time must increase as the controller gain decreases.
2. To most reliably avoid trips, the final control element should reach the appropriate extreme before the level gets to the high or low point that initiates a process trip.
Incorporating nonlinear relationships into the control equation can ensure the latter.
The nonlinear option for a PI control equation is implemented as follows:
Proportional mode. The proportional component of the output is a nonlinear function of the control error:
M = KC f(E) + MR
where M is controller output, %; KC is controller gain, %/%; E is control error, %, which equals the difference between the set point (SP) and process variable (PV), i.e., PV – SP for a direct acting controller and SP – PV for a reverse acting controller; and MR is controller output bias, %.
Integral mode. Although incorporating the nonlinear function into the integral mode also is possible, here we'll base the reset action on the control error (the same as for the linear control equation):
where TI is reset time, min; and MR,0 is the initial value for controller output bias, %.
Bumpless transfer calculations determine the value of MR,0, which is the initial condition on the reset integrator.
Despite basically unlimited possibilities, only two nonlinear relationships commonly appear:
• error gap or error deadband; and
• error squared.
ERROR GAP
In a true error deadband, the sensitivity within the deadband is zero. However, level control applications require a low sensitivity within the gap. Here, we'll call this relationship "error gap" and the value of the control error at which the sensitivity changes the "error deadband," EDB.
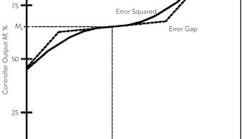
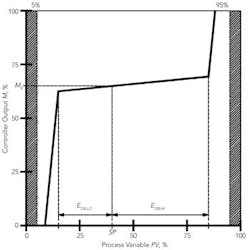
Figure 1 illustrates the desired relationship for the proportional mode. When PV equals SP, E is zero and M equals MR. As the PV deviates from SP in either direction, the sensitivity initially is low. But when the control error exceeds the value of the EDB, the sensitivity abruptly increases.
The relationship in Figure 1 involves three adjustable coefficients:
• Error deadband. Many implementations permit specifying separate values for PV > SP (the high deadband, EDBH) and PV < SP (the low deadband, EDBL). This is a desirable capability for level control applications.
• Controller gain within the gap. This sensitivity (KC,LO ) is applied when the control error is less than the error deadband.
• Controller gain outside the gap. This sensitivity (KC,HI) is applied when the control error exceeds the error deadband.
Using these coefficients, we can express the relationship illustrated in Figure 1 as:
M – MR = KC f(E)
= KC,LO E if |E| ≤ EDB
= KC,LO EDB + KC,HI[E - EDB] if E > EDB
= -KC,LO EDB + KC,LO [E + EDB] if E < -EDB
The following expression is also true:
Sensitivity = KC,LO if |E| ≤ EDB
= KC,HIif |E| > EDB
Most modern proportional-integral-derivative (PID) implementations permit specifying a computed value for the controller gain. This suggests the following implementation of the error gap relationship:
• Configure the PID control block as supplied by the manufacturer.
• Specify KC,LO for the controller gain if |E| ≤ EDB and KC,HIif |E| > EDB.
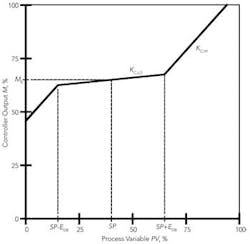
The success of this approach depends on how the PID is implemented. Where the position relationship is the basis for the PID calculations, the result is likely (but not necessarily) that illustrated in Figure 2. When the control error crosses the error deadband, an abrupt change occurs in the controller output — resulting in a "bump" to the process inappropriate for level control applications.
ERROR DEADBAND
For level control applications, the controller sensitivity within the gap must effectively respond to the "normal" variations in the feeds to the vessel. In effect, the sensitivity within the gap matches that of a customary linear PID control equation tuned without regard for the possibility of trips. The purpose of the nonlinearity within the control equation is to ensure the controller output reaches the appropriate extreme before either a high- or low-level process trip occurs.
At large values of the control error the error gap relationship gives a high sensitivity. However, this high sensitivity is not used to actually control the level. Instead, it serves to avoid a process trip by causing the controller to react quickly to drive the level back into the error gap.
For level control applications, the appropriate EDB value can be determined from the following (values in parentheses are from the previous articles):
• level set point (40%);
• vessel level at the location of the high level switch (95%); and
• vessel level at the location of the low level switch (5%).
When a discharge stream controls level its flow must be the maximum permitted, usually control valve fully open, before a high-level trip occurs. Similarly, the discharge flow must be the minimum permitted before a low-level trip occurs. Here we assume a valve fully closed — but some applications, such as pumping a starch slurry, cannot tolerate zero flows.
For the relationship in Figure 1, we can determine the controller output values for the PV values at each level switch:
High level (PV = 95%). The controller output attains 100% (control valve fully open) just before the vessel level reaches 95%. This meets the requirement for a fully open control valve should a process trip occur on high vessel level.
Low level (PV = 5%). The controller output is more than 50% at a vessel level of 5%. This does not meet the requirement for a fully closed control valve should a process trip occur on low vessel level.
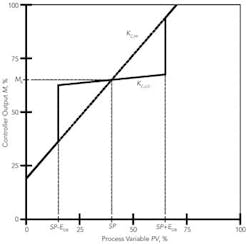
The error gap relationship illustrated in Figure 3 satisfies these requirements. The sensitivity within the error gap is the same as in Figure 1. The differences are:
• The controller gain outside the gap is 10 %/%. For this sensitivity a change of 10% in level would drive the output from 0% to 100% or vice versa.
• The error deadband high (PV > SP) is 45%, which makes the transition to the high sensitivity occur at a level of 85%. This provides a 10% change in level to drive the control valve fully open.
• The error deadband low (PV < SP) is 25%, which makes the transition to the high sensitivity occur at a level of 15%. This provides a 10% change in level to drive the control valve fully closed.
In Figure 3, the switch to the high sensitivity happens at a vessel level 10% on the safe side of the trip. This can be thought of as "elbow room." How much is required depends on how quickly the vessel level responds to a change in the flow in or out.
When using the error gap relationship for the proportional mode, the integral or reset mode serves the same purpose as in the customary PID control equation — to shift the controller output bias so the vessel level is controlled at its set point. In surge vessels with frequent changes in the flows in or out, the vessel level will not line out at the set point. However, it should vary around the set point, with approximately equal excursions above and below.
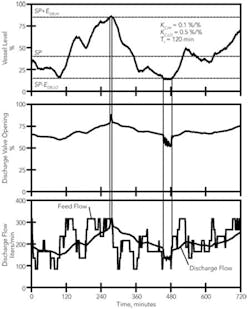
The previous article covered the response of the standard PI control equation for a controller gain of 0.4 %/% and a reset time of 120 min. The control equation provided a relatively smooth discharge flow without initiating any process trips on high level or low level.
Figure 4 shows the performance of the error gap control equation for a controller gain of 0.2 %/% within the gap and 10 %/% outside the gap. The discharge flow is even smoother. However, the vessel level occasionally makes brief excursions outside the gap — once above the upper deadband and once below the lower deadband. On each excursion the gain abruptly increases from 0.2 %/% to 10 %/%. This causes both the controller output and the discharge flow to change rapidly.
Rapid changes in the discharge flow have the desired effect of avoiding a process trip on high or low level. However, this is at the expense of an upset to the downstream unit. A process may tolerate occasional occurrences, especially if they are associated with a major upset or other abnormal event. However, if such excursions happen regularly you must increase the sensitivity within the gap.
ERROR SQUARED
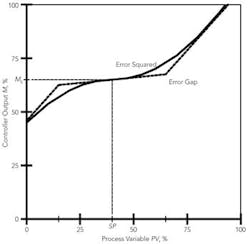
The term "signed error squared" (E2 < 0 if E < 0) more accurately reflects the nature of the relationship. To achieve this, we use |E| E in lieu of E2.
Figure 5 illustrates the relationship for the proportional mode. The dashed line is the error gap relationship from Figure 1. The two relationships are very similar, which suggests that if one will work, the other probably also will.
The proportional mode equation is:
M = KC |E/10| E + MR
Sensitivity = KC |E/10|
Incorporating the factor of 10 into the control equation gives the following behavior compared to the linear control equation:
E < 10%. The sensitivity is less.
E = 10%. The sensitivity is the same.
E > 10%. The sensitivity is greater.
Whereas the sensitivity of the error gap equation increases abruptly when the control error crosses the error deadband, the sensitivity of the error squared equation increases linearly with the control error.
The error squared relationship in Figure 5 provides only one adjustment, the controller gain. But for the same reasons that a high deadband and a low deadband are appropriate for the error gap equation, different sensitivities or gains are appropriate for the error squared control equation:
When PV > SP, use KC, HI as controller gain.
When PV < SP, use KC,LO as controller gain.
For level control applications, you can compute the values for the controller gains from the following values:
• normal value of the set point (40%);
• value of vessel level at the location of the high level switch (95%);
• value of vessel level at the location of the low level switch (5%); and
• typical value of controller output bias, MR (70%).
To ensure the final control element has been driven to an extreme before a trip occurs, you can compute the appropriate value of the controller gain from the error squared control equation:
KC = [(M – MR)/E] |10/E|
To determine KC,HI:
PV = 95% (high level trip point)
E = PV – SP = 55%
M = 100%
KC,HI= [(100% - 70%)/55%] |10%/55%| = 0.099 %%
To determine KC,LO:
PV = 5% (low level trip point)
E = PV – SP = -35%
M = 0%
KC,LO = [(0% - 70%)/-35%] |10%/-35%| = 0.57 %%
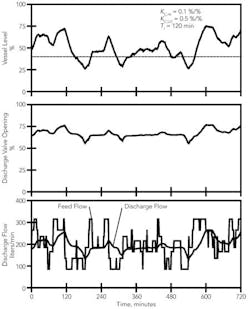
Figure 6 illustrates the performance of the error squared control equation for KC,HI= 0.1 %/% and KC,LO = 0.5 %/%. The low sensitivity above the set point makes the controller more tolerant of errors when PV > SP. Consequently, the vessel level is above its set point more than it is below it. Because of the higher sensitivity below the set point, rather rapid decreases occur in the controller output and the discharge flow when the vessel level drops below its set point. Reducing KC,LO would slow these changes — but would not ensure that the control valve is closed should the vessel level drop to the location of the low-level switch.
CECIL L. SMITH is president of Cecil L. Smith, Inc., Houston, Tex. E-mail him at [email protected]. This article is based on concepts from his book "Practical Process Control," published by John Wiley & Sons.