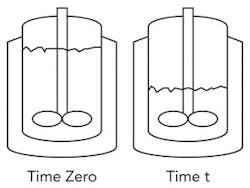
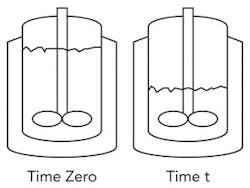
Batches often are concentrated by applying heat from steam in a jacket (or similar means). Common references such as Kern’s “Process Heat Transfer” [1] and “Perry’s Chemical Engineers’ Handbook” [2] cover transient heating and cooling, as does an earlier paper of mine [3]. However, their unsteady-state analyses treat wetted area as a constant, which clearly isn’t the case when a batch is boiled down.
Equally unhelpful, batch distillation literature primarily focuses on predicting component splits via vapor/liquid equilibrium and the Rayleigh Equation. No publication appears to offer a quick way to predict the time needed to distill or evaporate a batch to a desired concentration. This article introduces just such an equation.
Figure 1. Equation assumes liquid continues to contact the straight sides of the vessel.
Then, we calculate wetted areas per Eq. 4:
A0 = 23 + 4(98.25 - 9.89)/5 = 93.7 ft2
At = 23 + 4(82.48 - 9.89)/5 = 81.1 ft2
From Eq. 10, we determine characteristic time:
Θ = (62.3 × 5 × 1,036)/(4 × 50 × 165) = 9.78 hr
Using a rearrangement of Eq. 15 we get:
t = -Θ ln(At/A0) = - 9.78 ln(81.1/93.7) = -9.78 ln(0.8655) = 1.4 hr
So, the time needed to concentrate the batch from 735 gallons to 617 gallons is 1.4 hr.
MICHAEL J. GENTILCORE is Hazelwood, Mo.-based chemical process engineering manager for Mallinckrodt, a business unit of Covidien that will become a standalone company in 2013. E-mail him at [email protected]
NOMENCLATURE
A Area, ft2
D Tank diameter, ft
h Liquid height above head, ft
ΔH Heat of vaporization, Btu/lb
Q Heat transfer rate, Btu/hr
ΔT Temperature difference between jacket and process, °F
t Time, hr
U Overall heat transfer coefficient, Btu/(hr∙ft2∙°F)
V Volume, ft3
ρ Liquid density, lb/ft3
Θ Time constant (per Eq. 10), hr
Subscripts
0 Time zero
H Bottom head
S Straight side of vessel
T Total
t Time t
REFERENCES
1. Kern, D. Q., “Process Heat Transfer,” p. 624, McGraw-Hill, New York (1950) [still available in McGraw-Hill Classic Textbook Reissue Series].
2. Green, D. W. and Perry, R. H., “Perry’s Chemical Engineers’ Handbook,” 6th Ed., p. 10-38, McGraw Hill, New York (1997).
3. Gentilcore, M. J., “Estimate Heating and Cooling Times for Batch Reactors,” p. 41, Chem. Eng. Progress (March 2000).